4.four Measures of fundamental tendency
4.iv.three Calculating the mode

Text begins

When it'south unique, the mode is the value that appears the most often in a data set and information technology can exist used as a measure out of central tendency, like the median and mean. But sometimes, there is no way or there is more than ane mode.

There is no mode when all observed values appear the same number of times in a data set. There is more than one fashion when the highest frequency was observed for more one value in a data set. In both of these cases, the mode can't exist used to locate the centre of the distribution.

The way can be used to summarize categorical variables, while the mean and median can be calculated only for numeric variables. This is the main reward of the mode every bit a measure of central tendency. It's also useful for discrete variables and for continuous variables when they are expressed as intervals.

Hither are some examples of calculation of the mode for discrete variables.

Instance 1 – Number of points during a hockey tournament

During a hockey tournament, Audrey scored 7, 5, 0, 7, 8, 5, 5, four, 1 and v points in 10 games. Later summarizing the information in a frequency table, you tin hands see that the mode is 5 because this value appears the near often in the data set (four times). The mode can be considered a measure of central tendency for this data set considering it's unique.



Table iv.4.3.1
Number of games past the number of points scored
Table summary
This tabular array displays the results of Number of games by the number of points scored. The information is grouped by Number of points scored (appearing as row headers), Frequency (number of games) (appearing every bit cavalcade headers).
Number of points scored Frequency (number of games)
0   i
1   1
4   one
5   4
7   2
8   1
0 true zero or a value rounded to zero

Instance 2 – Number of points in 12 basketball games

During Marco's 12-game basketball flavour, he scored 14, 14, 15, sixteen, 14, 16, 16, eighteen, 14, 16, xvi and 14 points. Later summarizing the information in a frequency table, you lot tin can see that in that location are two modes in this information set: 14 and 16. Both values announced five times in the data set and v is the highest frequency observed. The fashion tin't exist used a measure of central tendency considering in that location is more than 1 mode. It's a bimodal distribution.



Table 4.4.3.two
Number of games by the number of points scored
Table summary
This table displays the results of Number of games by the number of points scored. The information is grouped by Number of points scored (appearing equally row headers), Frequency (number of games) (appearing every bit cavalcade headers).
Number of points scored Frequency (number of games)
14 v
xv 1
16 5
18 one

Example three – Number of touchdowns scored during football season

The following data set represents the number of touchdowns scored by Jerome in his high-school football season: 0, 0, 1, 0, 0, ii, 3, i, 0, 1, 2, 3, 1, 0. Permit's compare the mean, median and mode.
The sum of all values is xiv and there are 14 data points. This gives a mean of i. Because the number of values is even, the median is average between the data signal of rank vii and the information point of rank viii, after arranging the data set up in increasing order.



Table 4.4.3.3
Rank associated with each value of the number of touchdowns during football season
Tabular array summary
This table displays the results of Rank associated with each value of the number of touchdowns during football game flavour. The information is grouped past Rank (appearing as row headers), Number of touchdowns (appearing equally column headers).
Rank Number of touchdowns
1 0
2 0
3 0
4 0
five 0
half dozen 1
7 1
8 1
9 1
10 1
11 2
12 2
13 3
fourteen 3

Therefore, the median is equal to 1. Once the data has been summarized in a frequency table, y'all can see that the way is 0 because it is the value that appears the almost oftentimes (6 times).



Table 4.4.3.4
Number of games past the number of touchdowns
Table summary
This table displays the results of Number of games by the number of touchdowns. The information is grouped past Number of touchdowns (actualization as row headers), Frequency (actualization as column headers).
Number of touchdowns Frequency
0 6
1 four
2 2
3 2
0 truthful nothing or a value rounded to zero

In summary, in this case, the mean is one, the median is one and the way is 0.

The manner is not used as much for continuous variables because with this type of variable, it is likely that no value will appear more than than once. For example, if yous ask 20 people their personal income in the previous twelvemonth, it's possible that many will accept amounts of income that are very shut, merely that you volition never get exactly the same value for two people. In such case, information technology is useful to group the values in mutually exclusive intervals and to visualize the results with a histogram to place the modal-grade interval.

Case 4 – Pinnacle of people in the arena during a basketball game

Nosotros are interested in the tiptop of the people present in the arena during a basketball game. Tabular array iv.4.3.5 presents the number of people for 20-centimetre intervals of pinnacle.



Table 4.4.three.5
Number of people by top intervals
Table summary
This tabular array displays the results of Number of people by superlative intervals. The information is grouped past Top (in centimetres) (actualization every bit row headers), Frequency (number of people) (actualization as column headers).
Height (in centimetres) Frequency (number of people)
xx to 39 42
twoscore to 59 105
60 to 79 176
eighty to 99 230
100 to 119 214
120 to 139 168
140 to 159 363
160 to 179 480
180 to 200 170
200 to 219 eleven

Chart four.four.3.1 shows this information set every bit a histogram.

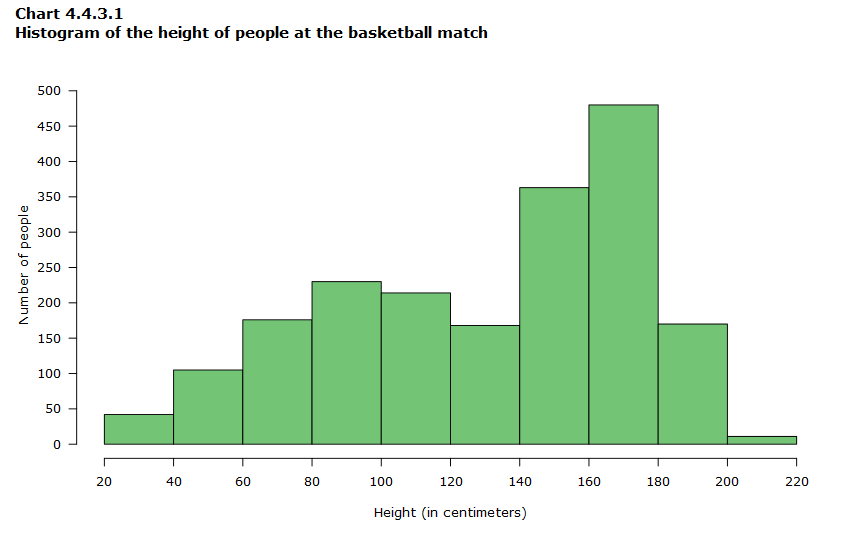
Chart 4.4.3.1 Histogram of the height of people at the basketball match

Information tabular array for Nautical chart 4.4.3.1

Data illustrated in this chart are the data from tabular array 4.iv.3.v.

Looking at the table and histogram, y'all can easily identify the modal-class interval, 160 to 179 centimetres, whose frequency is 480. You lot can also see that equally the height decreases from this interval, the frequency besides decreases for the interval 140 to 159 centimetres (363) and it continues to decrease for 120 to 139 centimetres (168), earlier starting to increase until the pinnacle reaches lxxx to 99 centimetres (230).

For categorical or discrete variables, multiple modes are values that attain the same frequency: the highest one observed. For continuous variables, all peaks of the distribution tin can exist considered modes fifty-fifty if they don't have the same frequency. The distribution for this case is bimodal, with a major manner corresponding to the modal-class interval 160 to 179 centimetres and a minor mode corresponding to the modal-course interval lxxx to 99 centimetres. The modal class shouldn't exist used as a measure of cardinal tendency, simply finding two modes gives us an indication that there could be 2 singled-out groups in the data that should be analyzed separately.


Report a problem on this page

Is something not working? Is there information outdated? Can't find what you're looking for?

Please contact us and allow usa know how we can help you.

Privacy observe

Date modified: